Hi Codeforces!
You may have used my CP Drills website earlier. It hasn't been hosted online for a while now. Many of you have messaged me so I decided to make its code available to the public here.
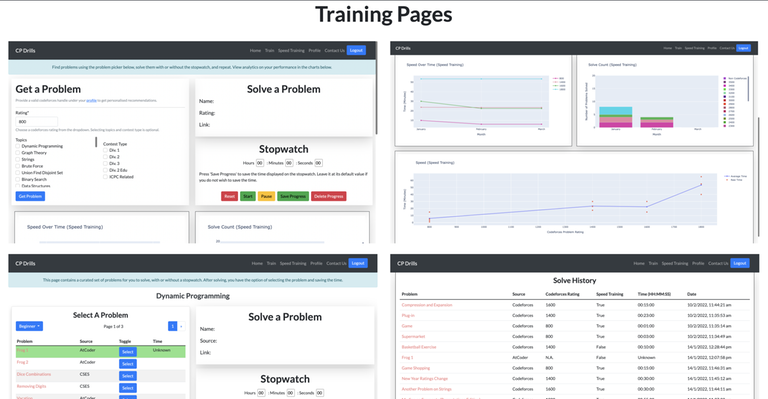
TLDR; you can choose problem tags and a rating and get a random problem from CF. You solve it against a stopwatch and then that metric gets added to different charts. Currently there's no support for automatically extracting the solve time, but that's something you might be interested in implementing for yourself!
Let me know if there are any issues in the installation instructions, and I'd love if you starred the repository if you found it helpful!